Ooh, we do love Friday Maths! Recently we started a statistical investigation to work out what numbers are most likely to occur if we rolled two dice together.
Firstly, we worked out that 7 was the most likely total to roll because you can get 7 by rolling 1+6, 2+5, 3+4, 4+3, 5+2 or 6+1. That means 7 has a better chance of being rolled than 8 because to roll an 8 you would need a 2+6, 3+5, 4+4, 5+3 or 6+2 which is less possible combinations.
You can see this in the chart below.
| Outcome |
Possible Combinations |
Total |
| 2 |
1+1 |
1 |
| 3 |
1+2, 2+1 |
2 |
| 4 |
1+3, 2+2, 3+1 |
3 |
| 5 |
1+4, 2+3, 3+2, 4+1 |
4 |
| 6 |
1+5, 2+4, 3+3, 4+2, 5+1 |
5 |
| 7 |
1+6, 2+5, 3+4, 4+3, 5+2, 6+1 |
6 |
| 8 |
2+6, 3+5, 4+4, 5+3, 6+2 |
5 |
| 9 |
3+6, 4+5, 5+4, 6+3 |
4 |
| 10 |
4+6, 5+5, 6+4 |
3 |
| 11 |
5+6, 6+5 |
2 |
| 12 |
6+6 |
1 |
You can see the rolling a 2 or a 12 are least likely but also equally unlikely.
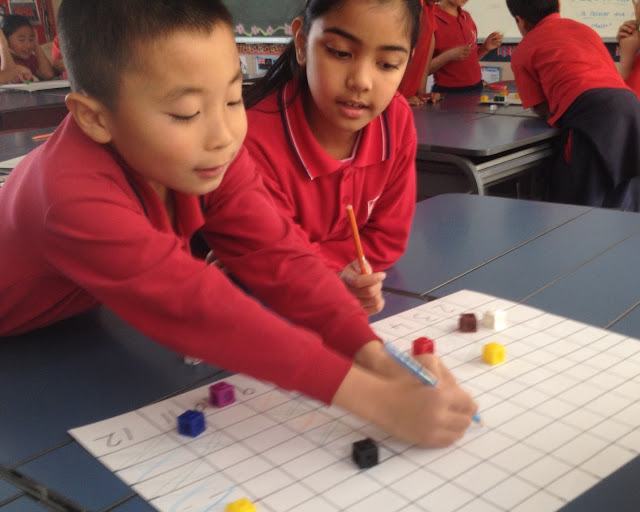
Next, we set up an random chance game to find out if this was true in real life. We wanted to see if we could beat the odds and have another number be the one that gets rolled more.
So we ruled up a page with 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, and 12 across the bottom and then ruled squares to make a track.
Then we used blocks to "race" to the finish line when we rolled our dice. Every time we rolled a number the block in that column "raced" forward one square. The first block to the edge of the page was the winner.
 |
| Most teams coloured in the squares to show the distribution of the outcomes. |
It was so fun! It was really interesting to see how even when you know there is a greater chance of something happening, it can never be guaranteed when you are relying on chance!
Here is one of the groups cheering on the winner which was not even number 8 but number 9!
It was really fab to see all the children really getting to understand how certain dice rolls are more likely or unlikely and how the statistical language of probability and chance can be applied to every day things, like playing board games.
So now we know that there is only a 1 in 36 chance of getting a double 1 or double 6 we can see why these are so hard to get when you really need them in a game like Ludo.